Home
School Exams
Scholarship Exams
OTI365 - NTSE - X Standard
NTSE Mental Ability Test - Arithmetical Reasoning Test Sample Questions with Answer

NTSE Mental Ability Test - Arithmetical Reasoning Test

Exam Duration: 45 Mins Total Questions : 30

1 )

If a clock takes seven seconds to strike seven, how long will it take to strike ten?

(a)
7 seconds
(b)
9 seconds
(c)
10 seconds
(d)
None of the above

Solution :

Clearly, seven strikes of a clock have 6 intervals while 10 strikes have 9 intervals
\(\therefore\) Required time = \((\frac{7}{6}\times9)\) = 10.5 seconds.

2 )

At the end of a business conference the ten people present all shake hands with each other once.How many handshakes will there be altogether?

(a)
20
(b)
45
(c)
55
(d)
90

Solution :

Clearly, total number of handshakes
= (9 + 8 + 7 + 6 + 5 + 4 + 3 + 2 + 1)
=45

3 )

Nitin's age was equal to square of some number last year and the following year it would be cube of a number. If again Nitin's age has to be equal to the cube of some number, then for how long he will have to wait?

(a)
10 years
(b)
38 years
(c)
39 years
(d)
64 years

Solution :

Clearly, we have to first find two numbers whose difference is 2 and of which the smaller one is a perfect square and the bigger one a perfect cube.
Such numbers are 25 and 27.
Thus, Nitin is now 26 years old. Since the next perfect cube after 27 is 64
So required time period = (64 - 26) years = 38 years

4 )

A number consists of two digits whose sum is 11. If 27 is added to the number, then the digits change their places. What is the number?

(a)
47
(b)
65
(c)
83
(d)
92

Solution :

Let the ten's digit be x. Then, unit's digit = (11-x).
So, number = 10x + (11 - x) = 9x + 11
Therefore (9x + 11) + 27 = 10 (11 - x) + x
\(\Leftrightarrow \) 9x + 38 = 110 - 9x 18x = 72, x = 4
Thus, ten's digit = 4 and unit's digit = 7
Hence, required number = 47

5 )

A monkey climbs 30 feet at the beginning of each hour and rests for a while when he slips back 20 feet befor he again starts climbing in the beginning of the next hour. If he begins his ascent at 8.00 a.m., at what time will he first touch a flag at 120 feet from the ground?

(a)
4 p.m.
(b)
5 p.m.
(c)
6 p.m.
(d)
None of these

Solution :

Net ascent of the monkey in 1 hour
= (30 - 20) feet = 10 feet
So, the monkey ascends 90 feet in 9 hours i.e. till 5 p.m.
Clearly, in the next 1 hour i.e. till 6 p.m. the monkey ascends remaining 30 feet to touch the flag.

6 )

A group of 1200 persons consisting of captains and soldiers is travelling in a train. For every 15 soldiers there is one captain. The number of captains in the group is

(a)
85
(b)
80
(c)
75
(d)
70

Solution :

Clearly, out of every 16 persons, there is one captain. So, the number of captains (1200/16) = 75

7 )

Ayush was born two years after his father's marriage.His mother is five years younger than his father but 20 years older than Ayush who is 10 years old. At what age did the father get married?

(a)
23 years
(b)
25 years
(c)
33 years
(d)
35 years

Solution :

Ayush's present age = 10 years
His mother's present age = (10 + 20) years
= 30 years
Ayush's father's present age = (30 + 5) years
= 35 years
Ayush's father's age at the time of Ayush's birth
= (35 - 10) years = 25 years
Therefore Ayush's father's age at the time of marriage
= (25 - 2) years = 23 years

8 )

The total number of digits used in numbering the pages of a book having 366 pages is

(a)
732
(b)
990
(c)
1098
(d)
1305

Solution :

Total number of digits = No. of digits in 1-digit page numbers + No. of digits in 2-digit page numbers + No.of digits in 3 - digit page numbers
= (1 x 9 + 2 x 90 + 3 x 267) = (9 + 180 + 801) = 990

9 )

Ravi's brother is 3 years senior to him. His father was 28 years of age when his sister was born while his mother was 26 years of age when he was born. If his sister was 4 years of age when his brother was born, what were the ages of Ravi's father and mother respectively when his brother was born?

(a)
32 years, 23 years
(b)
32 years, 29 years
(c)
35 years, 29 years
(d)
35 years, 33 years

Solution :

When Ravi's brother was born, let Ravi's father's age = x years and mother's age = y years.
Then, sister's age = (x - 28) years. So, x - 28 = 4 or x = 32.
Ravi's age = (y - 26) years. Age of Ravi's brother = (y - 26 + 3) years = (y - 23) years.
Now, when Ravi's brother was born, his age = 0 i.e.
y - 23 = 0 or y = 23.

10 )

A certain number of horses and an equal number of men are going somewhere. Half of the owners are on their horses' back while the remaining ones are walking along leading their horses. If the number of legs walking on the ground is 70, how many horses are there?

(a)
10
(b)
12
(c)
14
(d)
16

Solution :

Let number of horses = number of men = x
Then, number of legs = 4x + 2 x (x/2) = 5x
So, 5x = 70 or x = 14

11 )

When Rahul was born, his father was 32 years older than his brother and his mother was 25 years older than his sister. If Rahul's brother is 6 years older than him and his mother is 3 years younger than his father, how old was Rahul's sister when he was born?

(a)
7 years
(b)
10 years
(c)
14 years
(d)
19 years

Solution :

When Rahul was born, his brother's age = 6 years; his father's age = (6 + 32) years = 38 years, his mother's age = (38 - 3) years = 35 years; his sister's age = (35 - 25) years = 10 years

12 )

In a class, 20% of the members own only two cars each, 40% of the remaining own three cars each and the remaining members own only one car each. Which of the following statements is definitely true from the given statements?

(a)
Only 20% of the total members own three cars each.
(b)
48% of the total members own only one car each.
(c)
60% of the total members own at least two cars each.
(d)
80% of the total members own at least one car.

Solution :

Let total number of members be 100,
Then, number of members owning only 2 cars = 20
Number of members owning 3 cars = 40% of 80 = 32
Number of member» owning only 1 car = 100 - (20 + 32) = 48
Thus, 48% of the total members own one car each.

13 )

Three friends had dinner at a restaurant. When the bill was received, Amita paid 2/3 as much as Veena paid and Veena paid 1/2 as much as Tanya paid. What fraction of the bill did Veena pay?

(a)
1/3
(b)
3/11
(c)
12/13
(d)
5/8

Solution :

Let Tanya's share = Rs. x. Then, Veena's share = Rs. (x/2)
Total bill = Rs. (x + x/2 + x/3) = Rs. (11x/6)
Amita's share = Rs. (2/3 x x/2) = Rs. (x/3)
Therefore,
Required fraction = (x/2) x (6/11x) = 3/11.

14 )

What is the smallest number of ducks that could swim in this formation- two ducks in front of a duck two ducks behind a duck and a duck between two ducks?

(a)
3
(b)
5
(c)
7
(d)
9

Solution :

Clearly, the smallest such number is 3.

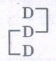
Three ducks can be arranged as shown above to satisfy all the three given conditions.

15 )

Today is Varun's birthday. One year, from today he will be twice as old as he was 12 years ago. How old is Varon today?

(a)
20 years
(b)
22 years
(c)
25 years
(d)
27 years

Solution :

Let Varun's age today = x years.
Then, Varon's age after 1 year = (x + 1) years
Therefore x + 1 = 2 (x - 12) \(\Leftrightarrow \) x + 1 = 2x - 24 \(\Leftrightarrow \) x = 25

16 )

A farmer built a fence around his square plot. He used 27 fence poles on each side of the square. How many poles did he need altogether?

(a)
100
(b)
104
(c)
108
(d)
None of these

Solution :

Since each pole at the corner of the plot is common to its two sides, so we have:
Total number of poles needed = 27 x 4 - 4 = 108 - 4 = 104.

17 )

If you write down all the numbers from 1 to 100 then how many times do you write 3?

(a)
11
(b)
18
(c)
20
(d)
21

Solution :

Clearly, from 1 to 100, there are ten numbers with 3 as the unit's digit - 3, 13, 23, 33,43, 53, 63, 73, 83, 93; and ten numbers with 3 as the ten's digit - 30, 31, 32, 33, 34, 35,36,37,38,39
So, required number = 10 + 10 = 20

18 )

Awaiter's salary consists of his salary and tips. During one week his tips were 5/4 of his salary. What fraction of his income came from tips?

(a)
4/9
(b)
5/4
(c)
5/8
(d)
5/9

Solution :

Let salary = Rs. x. Then tips = Rs. (5x/4)
Total income = Rs. (x + 5x/4) = Rs. (9x/4)
Therefore fraction = (5x/4) x (4/9x) = 5/9

19 )

A father is now three times as old as his son. Five years back, he was four times as old as his son. The age of the son (in years) is

(a)
12
(b)
15
(c)
18
(d)
20

Solution :

Let son's age be x years. Then, father's age = (3x) years
Five years ago, father's age = (3x - 5) years and son's age = (x - 5) years
So, 3x - 5 = 4 (x - 5) \(\Leftrightarrow \) 3x - 5 = 4x - 20 \(\Leftrightarrow \) x = 15

20 )

In a class, there are 18 boys who are over 160 cm tall. If these constitute three-fourths of the boys and the total number of boys is two-thirds of the total number of students in the class, what is the number of girls in the class?

(a)
6
(b)
12
(c)
18
(d)
24

Solution :

Let the number of boys be x. Then, (3/4)x = 18
or x = 18 x (4/3) = 24
If total number of students is y, then (2/3) y = 24
or y = 24 x (3/2) = 36
Therefore, the number of girls in the class = (36 - 24) = 12

21 )

There are deer and peacocks in a zoo. By counting heads they are 80. The number of their legs is 200. How many peacocks are there?

(a)
20
(b)
30
(c)
50
(d)
60

Solution :

Let x and y be the number of deer and peacock in the zoo respectively.
Then,
x + x = 80 ... (i) and 4x+ 2y= 200
or 2x + y = 100 ... (ii)
Solving (i) and (ii), we get) x = 20, y = 60

22 )

In a class of 60 students, the number of boys and girls participating in the annual sports is in the ratio 3:2 respectively. The number of girls not participating in the sports is 5 more than the number of boys not participating in the sports. If the number of boys participating in the sports is 15, then how many girls are there in the class?

(a)
20
(b)
25
(c)
30
(d)
Data inadequate

Solution :

Let the number of boys and girls participating in sports be 3x and 2x respectively.
Then, 3x= 15 or x= 5
So, the number of girls participating in sports = 2x = 10
Number of students not participating in sports = 60 - (15 + 10) = 35
Let number of boys not participating in sports be y
Then, number of girls not participating in sports = (35 - y)
Therefore (35 - y) = y + 5 2y 30 y = 15
So, number of girls not participating in sports = (35 - 15) = 20
Hence, total number of girls in the class = (10 + 20) = 30

23 )

A tailor had a number of shirt pieces to cut from a roll of fabric. He cut each roll of equal length into 10 pieces. He cut at the rate of 45 cuts a minute. How many rolls would be cut in 24 minutes?

(a)
32 rolls
(b)
54 rolls
(c)
108 rolls
(d)
120 rolls

Solution :

Number of cuts made to cut a roll into 10 pieces = 9
Therefore, required number ofrolls = (45 x 24)/9 = 120

24 )

In a garden, there are 10 rows and 12 columns of mango trees. The distance between the two trees is 2 metres and a distance of one metre is left from all sides of the boundary of the garden. The length of the garden is

(a)
20 m
(b)
22 m
(c)
24 m
(d)
26 m

Solution :

Each row contains 12 plants
There are 11 gapes between the two corner trees (11 x 2) metres and 1metre on each side is left.
Therefore, length = (22 + 2) m = 24 m

25 )

A is 3 years older to B and 3 years younger to C, while B and D are twins. How many years older is C to D?

(a)
2
(b)
3
(c)
6
(d)
12

Solution :

Since B and D are twins, so B = D
Now, A = B + 3 and A = C - 3.
Thus, B + 3 = C - 3; D + 3 = C - 3 C - D = 6

26 )

What is the product of all the numbers in the dial of a telephone?

(a)
1,58,480
(b)
1,59,450
(c)
1,59,480
(d)
None of these

Solution :

Since one of the numbers on the dial of a telephone is zero, so the product of all the numbers on it is 0.

27 )

A man has Rs. 480 in the denominations of one-rupee notes, five-rupee notes and ten-rupee notes. The number of notes of each denomination is equal. What is the total 6 number of notes that he has?

(a)
45
(b)
60
(c)
75
(d)
90

Solution :

Let number of notes of each denomination be x.
Then, x + 5x + 10x = 480 16x = 480 x = 30
Hence, total numbereof notes = 3x = 90

28 )

A number of friends decided to go on a picnic and planned to spend Rs. 96 on eatables. Four of them, however, did not turn up. As a consequence, the remaining ones had to contribute Rs. 4 each extra. The number of those who attended the picnic was

(a)
8
(b)
12
(c)
16
(d)
24

Solution :

Let the number of persons be x. Then,
96/(x - 4) - 96/x = 4
1/(x - 4) - 1/x = 4/96
[x - (x - 4)]/x (x - 4) = 1/24
x² - 4x - 96 = 0
(x - 12)(x + 8) = 0
x = 12
So, required number = x - 4 = 8

29 )

The total of the ages of Amar, Akbar and Anthony is 80 years. What was the total of their ages three years ago?

(a)
71 years
(b)
72 years
(c)
74 years
(d)
77 years

Solution :

Required sum.
= (80 - 3 x 3) years = (80 - 9) years = 71 years

30 )

I have a few sweets to be distributed. If I keep 2, 3 or 4 in a pack, I am left with one sweet. If I keep 5 in a pack, I am left with none. What is the minimum number of sweets I have to pack and distribute?

(a)
25
(b)
37
(c)
54
(d)
65

Solution :

Clearly, the required number would be such that it leaves a remainder of 1 when divided by 2, 3 or 4 and no remainder when divided by 5. Such a number is 25.